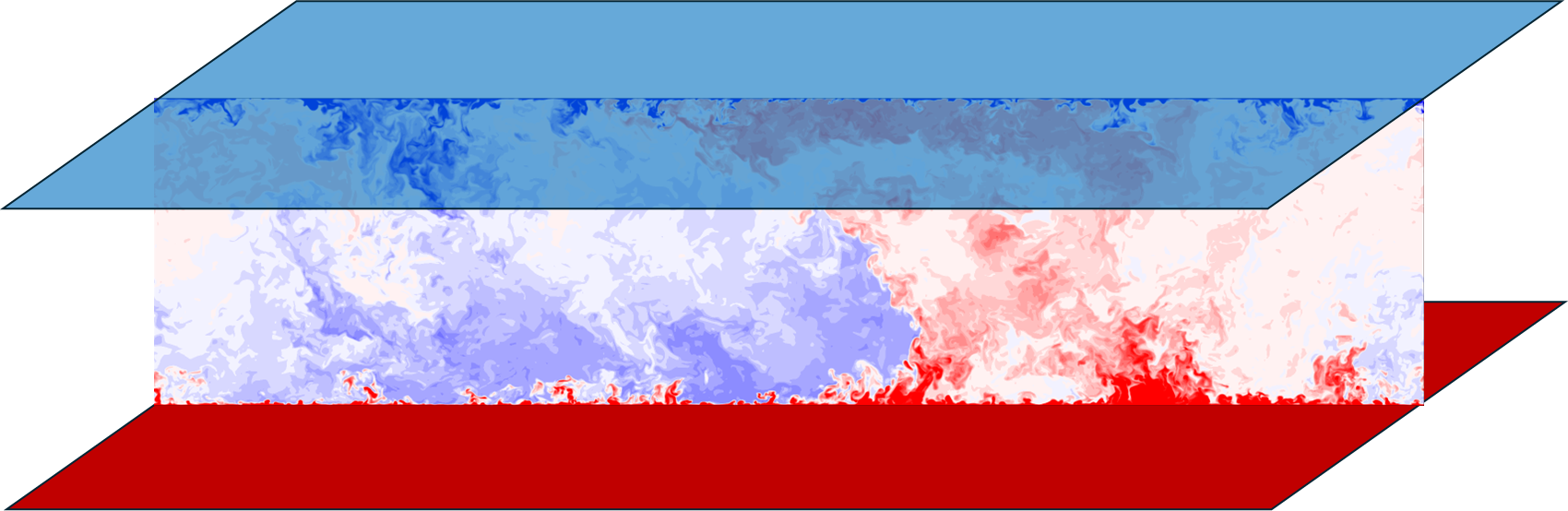
Rayleigh-Bénard Convection
Rayleigh-Bénard Convection DNS
Description
Rayleigh Benard Convection (RBC) is a benchmark fluid-dynamics problem for simulating natural thermal convection. It consists of a thin layer of fluid confined between a pair of parallel horizontal plates. The top plate is cooler than the bottom plate, and when this temperature difference is sufficiently high, a convective flow arises.This phenomenon can be simulated numerically by solving the incompressible Navier-Stokes equations under the Boussinesq approximation:
\[\frac{\partial \mathbf{u}^*}{\partial t^*} + \mathbf{u}^* \cdot \nabla \mathbf{u}^* = -\frac{1}{\rho_0} \nabla p^* + \nu \nabla^2 \mathbf{u}^* + \alpha g T^* \hat{\mathbf{z}}\] \[\frac{\partial T^*}{\partial t^*} + \mathbf{u}^* \cdot \nabla T^* = \kappa \nabla^2 T^*\] \[\nabla \cdot \mathbf{u}^* = 0\]Here, \(\mathbf{u}^*\), \(p^*\) and \(T^*\) are the velocity, pressure and temperature fields respectively. These quantities are in the dimensional form (including time, \(t^*\)). The length scales are non-dimensionalized with respect to the height of the domain, \(𝐻\). Similarly, the temperature field is non-dimensionalized by the temperature difference between the bottom and top plates, \(\Delta = 𝑇_𝑏 − 𝑇_𝑡\). This gives the free-fall velocity, \(𝑈_𝑓 = \sqrt{\alpha g \Delta 𝐻}\), which is used to non- dimensionalize the velocity field. The non-dimensional variables can therefore be written as:
\[\mathbf{u} = \frac{\mathbf{u}^*}{U_f} \quad ,\quad T = \frac{T^*}{\Delta} \quad ,\quad t = \frac{U_f t^*}{H} \quad ,\quad p = \frac{p^*-p_0}{\rho _0 U_f^2}\]where \(p_0\) and \(\rho _0\) are the reference pressure and density respectively. Since the DNS is performed with non-dimensional variables, the values of 𝑝0 and 𝜌0 are not set explicitly in the code. If necessary, they can be assumed to be 101.3 kPa and 1.2 kg/m3 respectively, as prescribed by the International Standard Atmosphere (ISA) at sea- level. Finally, we obtain the following non-dimensional equations for velocity and temperature which are solved in the DNS of RBC:
\[\frac{\partial \mathbf{u}}{\partial t} + \mathbf{u} \cdot \nabla \mathbf{u} = -\nabla p + \sqrt{Ra / Pr} \nabla^2 \mathbf{u} + T \hat{\mathbf{z}}\] \[\frac{\partial T}{\partial t} + \mathbf{u} \cdot \nabla T= \frac{1}{\sqrt{Ra Pr}} \nabla^2 T\] \[\nabla \cdot \mathbf{u} = 0\]The non-dimensional parameter, Rayleigh number (\(Ra\)), quantifies the degree of forcing imparted by buoyancy, whereas the Prandtl number (\(Pr\)) is the dimensionless ratio between the viscous and thermal diffusivities of the fluid:
\[Ra = \frac{\alpha g \Delta H^3}{\nu \kappa} \quad,\quad Pr = \frac{\nu}{\kappa}\]The present dataset is generated from DNS of RBC within a periodically extended Cartesian
box of aspect ratio \(\Gamma = L/H =4\), where L is the length of the box. All the simulations are performed with these fixed dimensions of 4 × 4 × 1. The DNS are performed using the GPU accelerated spectral element solver, NekRS [1], at a fixed \(Pr = 0.7\) and at \(10^5 \leq Ra \leq 10^9\).
Although the original simulations were performed on grids of increasingly finer resolutions [2], all fields have been interpolated to a uniform grid of size 2049 × 2049 × 1025 with a grid-spacing of 2h × 2h × h, where h is the grid spacing along the vertical 𝑧-axis. This
axis has a higher resolution to resolve the boundary layers properly. The interpolation was performed using spectral
element routines of NekRS itself to ensure maximum accuracy. There are 20 snapshots for each case.
Quick Info
Links to different cases
References
[1]. P. F. Fischer, S. Kerkemeier, M. Min, Y.-H. Lan, M. Phillips, T. Rathnayake, E. Merzari, A. Tomboulides, A. Karakus, N. Chalmers, and T. Warburton. a GPU-accelerated spectral element Navier–Stokes solver. Parallel Computing 114, 102982 (2022).
[2]. R. J. Samuel, M. Bode, J. D. Scheel, K. R. Sreenivasan and J. Schumacher. No sustained mean velocity in the boundary region of plane thermal convection. Journal of Fluid Mechanics 996, A49 (2024).